Tilman Enss group | Ruprecht-Karls-Universität Heidelberg
Team members
Dr. Moritz Drescher (Postdoc)
Dr. Keisuke Fujii (Postdoc)
Dr. Giacomo Gori (Postdoc)
Dr. Mrinal Sarkar (Postdoc)
Charalampos Pozoukidis (Master student)
Julius Tirpitz (Master student)
Former students and team members
B.Sc. Hagen Koch (Heidelberg 2024: Universality of one-dimensional Lévy flights)
M.Sc. Sarah Görlitz (Heidelberg 2024: Pattern Formation in driven Bose-Einstein Condensates)
M.Sc. Nikita Titov (Heidelberg 2023: Phase Transitions in Long-Range Spin Models; then PhD at U Trieste)
Dr. Félix Rose (postdoc; then Enseignant-chercheur at Cergy Paris Univ)
M.Sc. Eric Jacob (Heidelberg 2023: Anderson localization on long-range networks; then PhD at TU Vienna)
Dr. Giacomo Bighin (postdoc)
M.Sc. Daniel Arrufat Vicente (Heidelberg 2022: Quantum Brownian motion and the Bose polaron; then PhD at ETH Zurich)
Dr. Jeff Maki (visiting postdoc)
M.Sc. Marvin Syed (Heidelberg 2021: Dynamical quantum phase transition in the spherical model; then PhD at Cambridge University)
B.Sc. Timo Kleinbek (student RA)
B.Sc. Maximilian Kramer (Heidelberg 2021: Quantum Annealing within the LHZ Architecture)
B.Sc. Nikita Titov (Heidelberg 2021: Dynamics of two-dimensional Bose-Einstein condensates)
B.Sc. Julius Franke (Heidelberg 2021: Ultracold fermions periodically driven around unitarity)
Dr. Nicolò Defenu (postdoc; then Ass. Prof. at ETH Zurich)
B.Sc. Noel Cuadra Braatz (Heidelberg 2019: Quench and contact dynamics of two ultracold fermionic atoms)
Dr. Ana Paula Millán Vidal (Visiting postdoc)
B.Sc. Till Johann (Heidelberg 2019: Quench dynamics in strongly interacting trapped Fermi gases)
M.Sc. Hans Böhringer (Heidelberg 2019: Repulsive Fermi polarons in spin transport)
Andrea Colcelli (visiting PhD student)
M.Sc. Volker Karle (Heidelberg 2018: Binary Bose mixtures in 2D)
M.Sc. Martin Braß (Heidelberg 2017: Phase transitions and stability in resonant Bose-Fermi mixtures)
M.Sc. Sergej Trenkenschu (Heidelberg 2017: Quench dynamics of a Fermi gas)
B.Sc. Dominik Lorenz (Heidelberg 2016: Quench dynamics in the Hubbard model)
B.Sc. Daniel Issing (Heidelberg 2015: Bethe ansatz and quench dynamics for 1D integrable quantum systems)
B.Sc. Bruno Faigle-Cedzich (Heidelberg 2015: Shear viscosity of two-component Fermi gas)
Research interests
Our research focuses on many-body theory, mainly dynamics and
transport in strongly correlated quantum systems, ranging from
ultracold atomic gases to superconductors and quantum wires.
We work on the development of several modern and advanced
theoretical tools including the functional renormalization group
(fRG), Luttinger-Ward calculations, Hamiltonian flows, transfer-matrix DMRG and the
Lightcone RG.
- Transport bounds in strongly interacting Fermi gases
- Quantum scale anomaly and contact dynamics
- Impurity dynamics in Fermi gases and Bose-Einstein condensates
- Quantum magnets and the functional renormalization group (fRG)
- Complex networks and universality in fractional dimensions
- Quench dynamics in spin chains and many-body localization (MBL) using
the Light-cone renormalization group
group publications
articles on Google Scholar
Recent work
Hydrodynamic Attractor in Ultracold Atoms
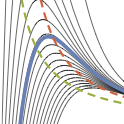
The hydrodynamic attractor is a concept that describes universal equilibration behavior in which systems lose microscopic details before hydrodynamics becomes applicable. We propose a setup to observe hydrodynamic attractors in ultracold atomic gases, taking advantage of the fact that driving the two-body s-wave scattering length causes phenomena equivalent to isotropic fluid expansions. We specifically consider two-component fermions with contact interactions in three dimensions and discuss their dynamics under a power-law drive of the scattering length in a uniform system, employing a hydrodynamic relaxation model. We analytically solve their dynamics and find the hydrodynamic attractor solution. Our results establish the cold atom systems as a new platform for exploring hydrodynamic attractors.
Keisuke Fujii and Tilman Enss,
arXiv:2404.12921
Particle and pair spectra for strongly correlated Fermi gases: A real-frequency solver
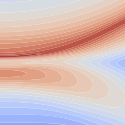
The strongly attractive Fermi gas in the BCS-BEC crossover is efficiently
described in terms of coupled fermions and fermion pairs, or molecules. We
compute the spectral functions of both fermions and pairs in the normal state
near the superfluid transition using a Keldysh formulation in real frequency.
The mutual influence between fermions and pairs is captured by solving the
self-consistent Luttinger-Ward equations: these include both the damping of
fermions by scattering off dressed pairs, as well as the decay of pair states
by dissociation into two dressed fermions. The pair spectra encode contact
correlations between fermions and form the basis for computing dynamical
response functions and transport properties.
Tilman Enss,
Phys. Rev. A 109, 023325 (2024)
Emergence of crystalline steady state in a driven superfluid
The spontaneous emergence of structures from initially homogenous systems belongs to the most striking topics in natural science. Systems driven into deeply nonlinear regimes are theoretically difficult to describe and can produce states that do not exist in equilibrium. We observe the emergence of a stable square lattice density modulation from an initially homogenous, two-dimensional, radially symmetric Bose-Einstein condensate when periodically driving the two-particle interaction. We show theoretically that this state can be understood as an attractive fixed point of coupled nonlinear amplitude equations, which result from phonon-phonon interactions. As a self-stabilized state characterized by spontaneously broken translational symmetry, our results establish a novel quantum material related to supersolids.
Nikolas Liebster, Marius Sparn, Elinor Kath, Keisuke Fujii, Sarah L. Görlitz, Tilman Enss, Helmut Strobel, and Markus K. Oberthaler,
arXiv:2309.03792
Medium-induced Interaction Between Impurities in a Bose-Einstein Condensate

We consider two heavy particles immersed in a Bose-Einstein condensate in three dimensions and compute their mutual interaction induced by excitations of the medium. For an ideal Bose gas, the induced interaction is Newtonian up to a shift in distance which depends on the coupling strength between impurities and Bosons. For a real BEC, we find that on short distances, the induced potential is dominated by three-body physics of a single Boson bound to the impurities, leading to an Efimov potential. At large distances of the order of the healing length, a Yukawa potential emerges instead. In particular, we find that both regimes are realized for all impurity-boson couplings and determine the corresponding crossover scales...
Moritz Drescher, Manfred Salmhofer, and Tilman Enss,
Phys. Rev. A 107, 063301 (2023)
Resonantly enhanced superconductivity mediated by spinor condensates
Achieving strong interactions in fermionic many-body systems is a major theme of research in condensed matter physics. It is well-known that interactions between fermions can be mediated through a bosonic medium, such as a phonon bath or Bose-Einstein condensate (BEC). Here, we show that such induced attraction can be resonantly enhanced when the bosonic medium is a two-component spinor BEC. The strongest interaction is achieved by tuning the boson-boson scattering to the quantum critical spinodal point of the BEC where the sound velocity vanishes. The fermion pairing gap and the superconducting critical temperature can thus be dramatically enhanced. We propose two experimental realizations of this scenario, with exciton-polariton systems in two-dimensional semiconductors and ultracold atomic Bose-Fermi mixtures.
Giacomo Bighin, Puneet A. Murthy, Nicolò Defenu, and Tilman Enss,
arXiv:2212.07419.
Universal scaling in fractional dimension

The concept of universality has shaped our understanding of many-body physics, but is mostly limited to homogenous systems. Here, we present a first study of universality on a non-homogeneous graph, the long-range diluted graph (LRDG). Its scaling theory is controlled by a single parameter, the spectral dimension ds, which plays the role of the relevant parameter on complex geometries. The graph under consideration allows us to tune the value of the spectral dimension continuously and find the universal exponents as continuous functions of the dimension. By means of extensive numerical simulations, we probe the scaling exponents of a simple instance of O(N) symmetric models on the LRDG showing quantitative agreement with the theoretical prediction of universal scaling in fractional dimensions...
Giacomo Bighin, Tilman Enss and Nicolò Defenu,
arXiv:2211.13302.
Transport in p-wave interacting Fermi gases
The scattering properties of spin-polarized Fermi gases are dominated
by p-wave interactions. Besides their inherent angular dependence,
these interactions differ from their s-wave counterparts as they
also require the presence of a finite effective range in order to
understand the low-energy properties of the system. In this article
we examine how the shear viscosity and thermal conductivity of a
three-dimensional spin-polarized Fermi gas in the normal phase
depend on the effective range and the scattering volume... In contrast to s-wave systems, p-wave scattering exhibits an additional resonance at weak attraction from a quasi-bound state at positive energies, which leads to a pronounced dip in the shear viscosity at specific temperatures.
Jeff Maki and Tilman Enss,
Phys. Rev. A 107, 023317 (2023).
Self-stabilized Bose polarons

The mobile impurity in a Bose-Einstein condensate (BEC) is a paradigmatic many-body problem. For weak interaction between the impurity and the BEC, the impurity deforms the BEC only slightly and it is well described within the Fröhlich model and the Bogoliubov approximation. For strong local attraction this standard approach, however, fails to balance the local attraction with the weak repulsion between the BEC particles and predicts an instability where an infinite number of bosons is attracted toward the impurity. Here we present a solution of the Bose polaron problem beyond the Bogoliubov approximation which includes the local repulsion between bosons and thereby stabilizes the Bose polaron even near and beyond the scattering resonance. We show that the Bose polaron energy remains bounded from below across the resonance and the size of the polaron dressing cloud stays finite. Our results demonstrate how the dressing cloud replaces the attractive impurity potential with an effective many-body potential that excludes binding...
Richard Schmidt and Tilman Enss,
SciPost Phys. 13, 054 (2022).
Complex networks with tuneable spectral dimension as a universality playground

Universality is one of the key concepts in understanding critical phenomena. However, for interacting inhomogeneous systems described by complex networks, a clear understanding of the relevant parameters for universality is still missing. Here we discuss the role of a fundamental network parameter for universality, the spectral dimension. For this purpose, we construct a complex network model where the probability of a bond between two nodes is proportional to a power law of the nodes' distances. By explicit computation we prove that the spectral dimension for this model can be tuned continuously from unity to infinity, and we discuss related network connectivity measures...
Ana P. Millán, Giacomo Gori, Federico Battiston, Tilman Enss, and
Nicolò Defenu,
Phys. Rev. Research 3, 023015 (2021).
Quench Dynamics of the Ideal Bose Polaron at Zero and Nonzero Temperatures

We give a detailed account of a stationary impurity in an ideal Bose-Einstein condensate, which we call the ideal Bose polaron, at both zero and nonzero temperatures and arbitrary strength of the impurity-boson coupling. The time evolution is solved exactly and it is found that, surprisingly, many of the features that have been predicted for the real BEC are already present in this simpler setting and can be understood analytically therein. We obtain explicit formulas for the time evolution of the condensate wave function at T=0 and of the one-particle density matrix at T>0. For negative scattering length, the system is found to thermalize even though the dynamics are perfectly coherent...
Moritz Drescher, Manfred Salmhofer, and Tilman Enss,
Phys. Rev. A 103, 033317 (2021).
Dynamical quantum phase transition in a bosonic system with long-range interactions
n this paper, we investigate the dynamical quantum phase transitions appearing in the Loschmidt echo and the time-dependent order parameter of a quantum system of harmonically coupled degenerate bosons as a function of the power-law decay σ of long-range interactions. Following a sudden quench, the nonequilibrium dynamics of this system are governed by a set of nonlinear coupled Ermakov equations. To solve them, we develop an analytical approximation valid at late times. Based on this approximation, we show that the emergence of a dynamical quantum phase transition hinges on the generation of a finite mass gap following the quench, starting from a massless initial state. The Loschmidt echo exhibits periodical nonanalytic cusps whenever the initial state has a vanishing mass gap and the final state has a finite mass gap.
Marvin Syed, Tilman Enss, and Nicolò Defenu,
Phys. Rev. B 103, 064306 (2021).
Interplay of spin waves and vortices in the two-dimensional XY model at small vortex-core energy
The Berezinskii-Kosterlitz-Thouless (BKT) mechanism describes universal vortex unbinding in many two-dimensional systems, including the paradigmatic XY model. However, most of these systems present a complex interplay between excitations at different length scales that complicates theoretical calculations of nonuniversal thermodynamic quantities. These difficulties may be overcome by suitably modifying the initial conditions of the BKT flow equations to account for noncritical fluctuations at small length scales. In order to systematically study the interplay between vortices and spin-wave excitations, we introduce a modified XY model with increased vortex fugacity. We present large-scale Monte-Carlo simulations of the spin stiffness and vortex density for this modified XY model and show that even at large vortex fugacity, vortex unbinding is accurately described by the nonperturbative functional renormalization group.
Ilaria Maccari, Nicolò Defenu, Lara Benfatto, Claudio Castellani, and Tilman Enss,
Phys. Rev. B 102, 104505 (2020).
Theory of a resonantly interacting impurity in a Bose-Einstein condensate

We investigate a Bose-Einstein condensate in strong interaction with a single impurity particle. While this situation has received considerable interest in recent years, the regime of strong coupling remained inaccessible to most approaches due to an instability in Bogoliubov theory arising near the resonance. We present a nonlocal extension of Gross-Pitaevskii theory that is free of such divergences and does not require the use of the Born approximation in any of the interaction potentials. We find a new dynamical transition regime between attractive and repulsive polarons, where an interaction quench results in a finite number of coherent oscillations in the density profiles of the medium and in the contact parameter before equilibrium is reached.
Moritz Drescher, Manfred Salmhofer, and Tilman Enss,
Phys. Rev. Research 2, 032011(R) (2020).
Scattering of two heavy Fermi polarons: resonances and quasibound states
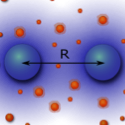
Impurities in a Fermi sea, or Fermi polarons, experience a Casimir interaction induced by quantum fluctuations of the medium. When there is short-range attraction between impurities and fermions, also the induced interaction between two impurities is strongly attractive at short distance and oscillates in space for larger distances. We theoretically investigate the scattering properties and compute the scattering phase shifts and scattering lengths between two heavy impurities in an ideal Fermi gas at zero temperature. While the induced interaction between impurities is weakly attractive for weak impurity-medium interactions, we find that impurities strongly and attractively interacting with the medium exhibit resonances in the induced scattering with a sign change of the induced scattering length and even strong repulsion...
Tilman Enss, Binh Tran, Michael Rautenberg, Manuel Gerken, Eleonora Lippi, Moritz Drescher, Bing Zhu, Matthias Weidemüller, and Manfred Salmhofer,
Phys. Rev. A 102, 063321 (2020).
Quantum critical thermal transport in the unitary Fermi gas
Strongly correlated systems are often associated with an underlying quantum critical point which governs their behavior in the finite-temperature phase diagram. Their thermodynamical and transport properties arise from critical fluctuations and follow universal scaling laws. Here, we develop a microscopic theory of thermal transport in the quantum critical regime expressed in terms of a thermal sum rule and an effective scattering time. We explicitly compute the characteristic scaling functions in a quantum critical model system, the unitary Fermi gas. Moreover, we derive an exact thermal sum rule for heat and energy currents and evaluate it numerically using the nonperturbative Luttinger-Ward approach. For the thermal scattering times we find a simple quantum critical scaling form. Together, the sum rule and the scattering time determine the heat conductivity, thermal diffusivity, Prandtl number, and sound diffusivity from high temperatures down into the quantum critical regime. The results provide a quantitative description of recent sound attenuation measurements in ultracold Fermi gases.
Bernhard Frank, Wilhelm Zwerger, and Tilman Enss,
Phys. Rev. Research 2, 023301 (2020).
Sound propagation and quantum limited damping in a two-dimensional Fermi gas
PRL Editors' suggestion

Strongly interacting two-dimensional Fermi systems are one of the great remaining challenges in many-body physics due to the interplay of strong local correlations and enhanced long-range fluctuations. Here, we probe the thermodynamic and transport properties of a 2D Fermi gas across the BEC-BCS crossover by studying the propagation and damping of sound modes. We excite particle currents by imprinting a phase step onto homogeneous Fermi gases trapped in a box potential and extract the speed of sound from the frequency of the resulting density oscillations. We measure the speed of sound across the BEC-BCS crossover and compare the resulting dynamic measurement of the equation of state both to a static measurement based on recording density profiles and to Quantum Monte Carlo calculations and find reasonable agreement between all three. We also measure the damping of the sound mode, which is determined by the shear and bulk viscosities as well as the thermal conductivity of the gas. We find that the damping is minimal in the strongly interacting regime and the diffusivity approaches the universal quantum bound ℏ/m of a perfect fluid.
Markus Bohlen, Lennart Sobirey, Niclas Luick,
Hauke Biss, Tilman Enss, Thomas Lompe, and Henning Moritz,
Phys. Rev. Lett. 124, 240403 (2020).
Bulk viscosity and contact correlations in attractive Fermi gases
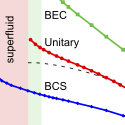
The bulk viscosity determines dissipation during hydrodynamic expansion. It vanishes in scale invariant fluids, while a nonzero value quantifies the deviation from scale invariance. For the dilute Fermi gas the bulk viscosity is given exactly by the correlation function of the contact density of local pairs. As a consequence, scale invariance is broken purely by pair fluctuations. These fluctuations give rise also to logarithmic terms in the bulk viscosity of the high-temperature nondegenerate gas. For the quantum degenerate regime I report numerical Luttinger-Ward results for the contact correlator and the dynamical bulk viscosity throughout the BEC-BCS crossover. The ratio of bulk to shear viscosity ζ/η is found to exceed the kinetic theory prediction in the quantum degenerate regime. Near the superfluid phase transition the bulk viscosity is enhanced by critical fluctuations and has observable effects on dissipative heating, expansion dynamics and sound attenuation.
Tilman Enss,
Phys. Rev. Lett. 123, 205301 (2019).
Published in Science:
Quantum scale anomaly and spatial coherence in a 2D Fermi superfluid
Quantum anomalies are violations of classical scaling symmetries caused by divergences that appear in the quantization of certain classical theories. Although they play a prominent role in the quantum field theoretical description of many-body systems, their influence on experimental observables is difficult to discern. In this study, we discovered a distinctive manifestation of a quantum anomaly in the momentum-space dynamics of a two-dimensional (2D) Fermi superfluid of ultracold atoms. The measured pair momentum distributions of the superfluid during a breathing mode cycle exhibit a scaling violation in the strongly interacting regime. We found that the power-law exponents that characterize long-range phase correlations in the system are modified by the quantum anomaly, emphasizing the influence of this effect on the critical properties of 2D superfluids.
P. A. Murthy, N. Defenu, L. Bayha, M. Holten, P. M. Preiss, T. Enss, and S. Jochim,
Science 365, 268-272 (2019).
Press release of Heidelberg University
Berezinskii-Kosterlitz-Thouless paired phase in coupled XY models
We study the effect of a linear tunneling coupling between two-dimensional systems, each separately exhibiting the topological Berezinskii-Kosterlitz-Thouless (BKT) transition. In the uncoupled limit, there are two phases: one where the one-body correlation functions are algebraically decaying and the other with exponential decay. When the linear coupling is turned on, a third BKT-paired phase emerges, in which one- body correlations are exponentially decaying, while two-body correlation functions exhibit power-law decay. We perform numerical simulations in the paradigmatic case of two coupled XY models at finite temperature, finding evidences that for any finite value of the interlayer coupling, the BKT-paired phase is present. We provide a picture of the phase diagram using a renormalization group approach.
G. Bighin, N. Defenu, I. Nándori, L. Salasnich, and A. Trombettoni,
Phys. Rev. Lett. 123, 100601 (2019).
Dynamical criticality and domain-wall coupling in long-range Hamiltonians
Dynamical quantum phase transitions hold a deep connection to the underlying equilibrium physics of the quench Hamiltonian. In a recent study [J. C. Halimeh et al., arXiv:1810.07187] it has been numerically demonstrated that the appearance of anomalous cusps in the Loschmidt return rate coincides with the presence of bound domain walls in the spectrum of the quench Hamiltonian. Here we consider transverse-field Ising chains with power-law and exponentially decaying interactions, and show that by removing domain-wall coupling via a truncated Jordan-Wigner transformation onto a Kitaev chain with long-range hopping and pairing, anomalous dynamical criticality is no longer present. This indicates that bound domain walls are necessary for anomalous cusps to appear in the Loschmidt return rate. We also calculate the dynamical phase diagram of the Kitaev chain with long-range hopping and pairing, which in the case of power-law couplings is shown to exhibit rich dynamical criticality including a doubly critical dynamical phase.
N. Defenu, T. Enss, and J. C. Halimeh,
Phys. Rev. B 100, 014434 (2019).
Real-space dynamics of attractive and repulsive polarons in Bose-Einstein condensates
We investigate the formation of a Bose polaron when a single
impurity in a Bose-Einstein condensate is quenched from a
non-interacting to an attractively interacting state in the vicinity
of a Feshbach resonance. We use a beyond-Fröhlich Hamiltonian
to describe both sides of the resonance and a coherent-state
variational ansatz to compute the time evolution of boson density
profiles in position space. We find that on the repulsive side of
the Feshbach resonance, the Bose polaron performs long-lived
oscillations, which is surprising given that the two-body problem
has only one bound state coupled to a continuum. They arise due to
interference between multiply occupied bound states and therefore
can be only found with many-body approaches such as the
coherent-state ansatz. Furthermore, an impurity
introduced at non-zero velocity on the repulsive
side is periodically slowed down or even arrested before
speeding up again.
M. Drescher, M. Salmhofer, and T. Enss,
Phys. Rev. A 99, 023601 (2019).
Universal Spin Transport and Quantum Bounds for Unitary Fermions
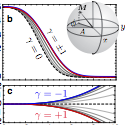
We review recent advances in experimental and theoretical understanding of spin transport in strongly interacting Fermi gases. The central new phenomenon is the observation of a lower bound on the (bare) spin diffusivity in the strongly interacting regime. Transport bounds are of broad interest for the condensed matter community, with a conceptual similarity to observed bounds in shear viscosity and charge conductivity. We discuss the formalism of spin hydrodynamics, how dynamics are parameterized by transport coefficients, the effect of confinement, the role of scale invariance, the quasi-particle picture, and quantum critical transport. We conclude by highlighting open questions, such as precise theoretical bounds, relevance to other phases of matter, and extensions to lattice systems.
T. Enss and J. H. Thywissen,
invited review for Annu. Rev. Condens. Matter Phys. 10, 85-106 (2019).
High-temperature pairing in a strongly interacting two-dimensional Fermi gas
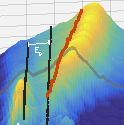
The nature of the normal phase of strongly correlated fermionic systems is an outstanding question in quantum many-body physics. We use spatially resolved radio-frequency spectroscopy to measure pairing energy of fermions across a wide range of temperatures and interaction strengths in a two-dimensional gas of ultracold fermionic atoms. We observed many-body pairing at temperatures far above the critical temperature for superfluidity. In the strongly interacting regime, the pairing energy in the normal phase considerably exceeds the intrinsic two-body binding energy of the system and shows a clear dependence on local density. This implies that pairing in this regime is driven by many-body correlations, rather than two-body physics. Our findings show that pairing correlations in strongly interacting two-dimensional fermionic systems are remarkably robust against thermal fluctuations.
Puneet
A. Murthy, Mathias Neidig, Ralf Klemt, Luca Bayha, Igor Boettcher,
Tilman Enss, Marvin Holten, Gerhard Zürn, Philipp M. Preiss,
and Selim Jochim,
Science 359, 452-455 (2018).
Exploring the ferromagnetic behaviour of a repulsive Fermi gas through spin dynamics
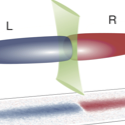
Ferromagnetism is a manifestation of strong repulsive interactions between itinerant fermions in condensed matter. Whether short-ranged repulsion alone is sufficient to stabilize ferromagnetic correlations in the absence of other effects, such as peculiar band dispersions or orbital couplings, is, however, unclear. Here, we investigate ferromagnetism in the minimal framework of an ultracold Fermi gas with short-range repulsive interactions tuned via a Feshbach resonance...
G. Valtolina,
F. Scazza, A. Amico, A. Burchianti, A. Recati, T. Enss, M. Inguscio,
M. Zaccanti, and G. Roati,
Nature Phys. 13, 704
(2017).
Observation of quantum-limited spin transport in strongly interacting two-dimensional Fermi gases
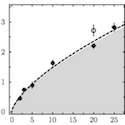
We measure the transport and thermodynamic properties of two-dimensional ultracold Fermi gases through their demagnetization in a magnetic field gradient. Using a phase-coherent spin-echo sequence, we are able to distinguish bare spin diffusion from the Leggett-Rice effect, in which demagnetization is slowed by the precession of spin current around the local magnetization. When the two-dimensional scattering length is comparable to the inverse Fermi wave vector, we find that the bare transverse spin diffusivity reaches a minimum of 1.7(6)ℏ/m...
C. Luciuk,
S. Smale, F. Böttcher, H. Sharum, B. A. Olsen, S. Trotzky,
T. Enss, and J. H. Thywissen,
Phys. Rev. Lett. 118, 130405 (2017).
slides of talk in Trieste, 2017.

We report the experimental measurement of the equation of state of a two-dimensional Fermi gas with attractive s-wave interactions throughout the crossover from a weakly coupled Fermi gas to a Bose gas of tightly bound dimers as the interaction strength is varied. We demonstrate that interactions lead to a renormalization of the density of the Fermi gas by several orders of magnitude. We compare our data near the ground state and at finite temperature with predictions for both fermions and bosons from quantum Monte Carlo simulations and Luttinger-Ward theory. Our results serve as input for investigations of close-to-equilibrium dynamics and transport in the two-dimensional system.
Boettcher,
L. Bayha, D. Kedar, P. A. Murthy, M. Neidig, M. G. Ries, A. N. Wenz,
G. Zürn, S. Jochim, and T. Enss,
Phys. Rev. Lett. 116,
045303 (2016).
Viewpoint in Physics:
Journey from Classical to Quantum in Two Dimensions, by Meera
M. Parish.
Ultrakalte
Atome in zwei Dimensionen (article in German, Phys. Unserer Zeit 47, 113 (2016)).
Nonlinear spin diffusion and spin rotation in a trapped Fermi gas
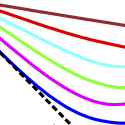
Transverse spin diffusion in a polarized, interacting Fermi gas leads to the Leggett-Rice effect, where the spin current precesses around the local magnetization. With a spin-echo sequence both the transverse diffusivity and the spin-rotation parameter γ are obtained; the sign of γ reveals the repulsive or attractive character of the effective interaction. In a trapped Fermi gas the spin diffusion equations become nonlinear, and their numerical solution exhibits an inhomogeneous spin state even at the spin echo time. While the microscopic diffusivity and γ increase at weak coupling, their apparent values inferred from the trap-averaged magnetization saturate in agreement with a recent experiment for a dilute ultracold Fermi gas.
Tilman Enss, Phys. Rev. A 91, 023614 (2015).
Observation of the Leggett-Rice effect in a unitary Fermi
gas
PRL Editors' suggestion
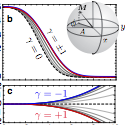
We observe that the diffusive spin current in a strongly interacting degenerate Fermi gas of 40K precesses about the local magnetization. As predicted by Leggett and Rice, precession is observed both in the Ramsey phase of a spin-echo sequence, and in the nonlinearity of the magnetization decay. At unitarity, we measure a Leggett-Rice parameter γ=1.08(9) and a bare transverse spin diffusivity D
0⊥=2.3(4)ℏ/m for a normal-state gas initialized with full polarization and at one fifth of the Fermi temperature, where m is the atomic mass. For a unitary gas, γ→0 as temperature is increased to the Fermi temperature. Tuning the scattering length a, we find that a sign change in γ occurs in the range 0<(kFa)
-1≤1.3, where kF is the Fermi momentum. We discuss how γ reveals the effective interaction strength of the gas, such that a change in γ indicates a switching of branch, between a repulsive and an attractive Fermi gas.
S. Trotzky, S. Beattie, C. Luciuk, S. Smale, A. B. Bardon, T. Enss,
E. Taylor, S. Zhang, and J. H. Thywissen,
Phys. Rev. Lett. 114, 015301 (2015).
Purification and many-body localization in cold atomic gases
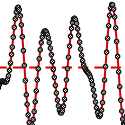
We propose to observe many-body localization in cold atomic gases by realizing a Bose-Hubbard chain with binary disorder and studying its non-equilibrium dynamics. In particular, we show that measuring the difference in occupation between even and odd sites, starting from a prepared density-wave state, provides clear signatures of localization. As hallmarks of the many-body localized phase we confirm, furthermore, a logarithmic increase of the entanglement entropy in time and Poissonian level statistics. Our numerical density-matrix renormalization group calculations for infinite system size are based on a purification approach which allows to perform the disorder average exactly, thus producing data without any statistical noise, and with maximal simulation times of up to a factor 10 longer than in the clean case.
Felix Andraschko, Tilman Enss, and Jesko Sirker, Phys. Rev. Lett. 113, 217201 (2014).
Universal equation of state and pseudogap in the two-dimensional Fermi gas
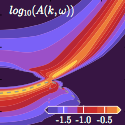
We determine the thermodynamic properties and the spectral function for a homogeneous two- dimensional Fermi gas in the normal state using the Luttinger-Ward, or self-consistent T-matrix, approach. The density equation of state deviates strongly from that of the ideal Fermi gas even for moderate interactions, and our calculations suggest that temperature has a pronounced effect on the pressure in the crossover from weak to strong coupling, consistent with recent experiments. We also compute the superfluid transition temperature for a finite system in the crossover region. There is a pronounced pseudogap regime above the transition temperature: the spectral function shows a Bogoliubov-like dispersion with backbending, and the density of states is significantly suppressed near the chemical potential. The contact density at low temperatures increases with interaction and compares well with both experiment and zero-temperature Monte Carlo results.
Marianne Bauer, Meera M. Parish, and Tilman Enss, Phys. Rev. Lett. 112, 135302 (2014).
Damping of the quadrupole mode in a two-dimensional Fermi gas
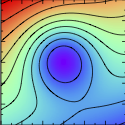
In a recent experiment [E. Vogt et al., Phys. Rev. Lett. 108, 070404 (2012)], quadrupole and breathing modes of a two-dimensional Fermi gas were studied. We model these collective modes by solving the Boltzmann equation via the method of phase-space moments up to fourth order, including in-medium effects on the scattering cross section. In our analysis, we use a realistic Gaussian potential deformed by the presence of gravity and magnetic field gradients. We conclude that the origin of the experimentally observed damping of the quadrupole mode, especially in the weakly interacting (or even non-interacting) case, cannot be explained by these mechanisms.
Silvia Chiacchiera, Dany Davesne, Tilman Enss, and Michael Urban, Phys. Rev. A 88, 053616 (2013).
M. Urban, S. Chiacchiera, D. Davesne,
T. Enss, and P.-A. Pantel, J. Phys.: Conf. Ser. 497, 012028 (2014).
Quantum critical transport in the unitary Fermi gas
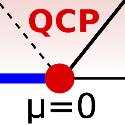
The thermodynamic and transport properties of the unitary Fermi gas
at finite temperature T are governed by a quantum critical point at
T=0 and zero density. We compute the universal shear viscosity to
entropy ratio η/s in the high-temperature quantum critical regime
T≫|μ| and find that this strongly coupled quantum fluid comes
close to perfect fluidity η/s=ℏ/4π
kB. Using a
controlled large-N expansion we show that already at the first
non-trivial order the equation of state and the Tan contact density
C agree well with the most recent experimental measurements and
theoretical Luttinger-Ward and Bold Diagrammatic Monte Carlo
calculations.
Tilman
Enss, Phys. Rev. A 86, 013616 (2012).
slides of
talk at ERG 2012 conference
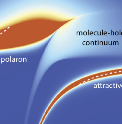
Excitation spectra and rf-response near the polaron-to-molecule
transition from the functional renormalization group
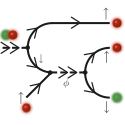
A light impurity in a Fermi sea undergoes a transition from a
polaron to a molecule for increasing interaction. We develop a
new method to compute the spectral functions of the polaron
and molecule in a unified framework based on the functional
renormalization group with full self-energy feedback. We
discuss the energy spectra and decay widths of the attractive
and repulsive polaron branches as well as the molecular bound
state and confirm the scaling of the excited state decay rate
near the transition. The quasi-particle weight of the polaron
shifts from the attractive to the repulsive branch across the
transition, while the molecular bound state has a very small
residue characteristic for a composite particle. We propose an
experimental procedure to measure the repulsive branch in a
Li6 Fermi gas using rf-spectroscopy and calculate the
corresponding spectra.
Richard Schmidt and Tilman Enss, Phys. Rev. A 83, 063620 (2011).
slides of talk
at ERG 2014 conference
Konzept: Carmen
Enss, Büro für Denkmalforschung und Denkmalpflege